Enthalpies of Formation
Related Examples and Practice Problems
Topic Summary & Highlights
and Help Videos
Core Concept
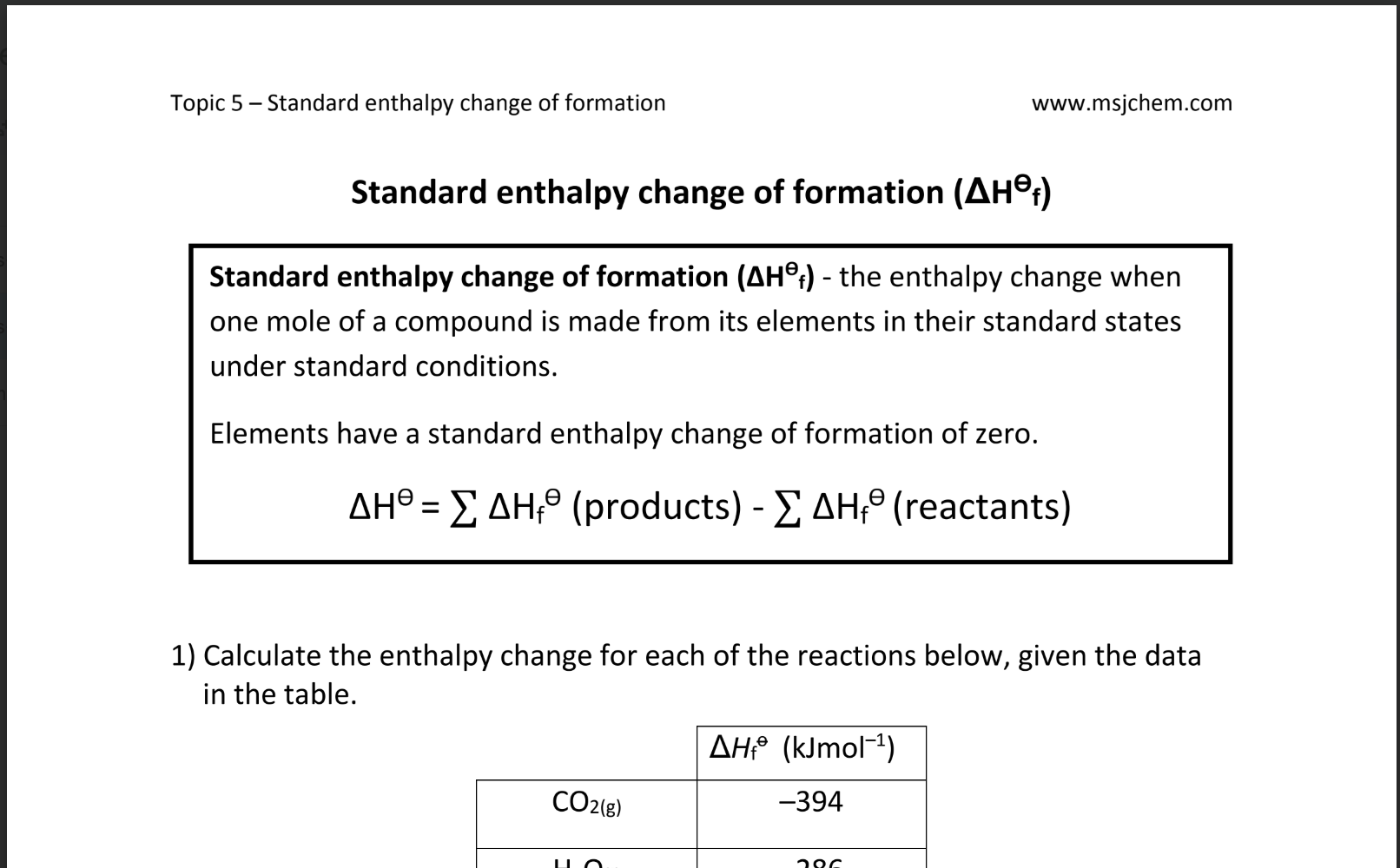
Enthalpy of Formation ($\Delta H_f^\circ$) is the enthalpy change when 1 mole of a compound is formed from its elements in their standard states under standard conditions (298 K and 1 atm). Standard enthalpies of formation are essential for calculating the enthalpy changes of reactions.
Practice Tips
Use Standard Enthalpies: Always use $\Delta H_f^\circ$ values from standard tables to ensure accuracy.
Check Physical States: Ensure each substance’s physical state (solid, liquid, gas) matches its enthalpy of formation, as $\Delta H_f^\circ$ values vary by state.
Remember Elements Have $Delta H_f^\circ = 0$: Pure elements in their standard states have a formation enthalpy of zero.
Multiply by Stoichiometric Coefficients: Don’t forget to multiply each $\Delta H_f^\circ$∘ by its coefficient from the balanced chemical equation.
Calculating Enthalpy of a Reaction Using Enthalpies of Formation
The enthalpy change of a reaction can be calculated using the enthalpies of formation of the reactants and products:
$\Delta H_{\text{reaction}}^\circ = \sum \Delta H_f^\circ(\text{products}) - \sum \Delta H_f^\circ(\text{reactants})$
Identify the Products and Reactants:
Write the balanced chemical equation for the reaction.
Find the Enthalpies of Formation:
Look up the $\Delta H_f^\circ$ values for each reactant and product in a table.
Multiply by Coefficients:
Multiply each $\Delta H_f^\circ$ value by its coefficient in the balanced equation.
Subtract the Sum of Reactants from Products:
Sum the enthalpies of formation for the products and reactants separately, then subtract the total for the reactants from the total for the products.
Key Concepts
Standard Enthalpy of Formation ($\Delta H_f^\circ$):
The enthalpy change when 1 mole of a compound is formed from its elements in their most stable forms at 1 atm pressure and 298 K.
Symbol: $\Delta H_f^\circ$ (the degree symbol $\circ$∘ denotes standard conditions).
Measured in kilojoules per mole (kJ/mol).
Standard State:
Elements are in their most stable physical forms at 1 atm and 298 K (e.g., $\text{O}_2$ for oxygen, $\text{N}_2$ for nitrogen, and graphite for carbon).
Enthalpy of Formation of Elements:
The standard enthalpy of formation of any pure element in its standard state is zero.
Example: $\Delta H_f^\circ$ for $\text{O}_2\text{(g)}$ and $\text{N}_2\text{(g)}$ is zero.
Applications:
Enthalpies of formation are used to calculate the enthalpy change for chemical reactions.
Hess’s Law and standard enthalpies of formation can be combined to find $\Delta H_{\text{reaction}}^\circ$.
Example Problem
Calculate the enthalpy change (ΔHrxn) for the combustion of methane ($\text{CH}_4$) given the following reaction:
$\text{CH}_4(g) + 2\text{O}_2(g) \rightarrow \text{CO}_2(g) + 2\text{H}_2\text{O}(l)$
Use the standard enthalpies of formation ($\Delta H^\circ_f$):
$\Delta H^\circ_f (\text{CH}_4(g)) = -74.8 \, \text{kJ/mol}$
$\Delta H^\circ_f (\text{O}_2(g)) = 0 \, \text{kJ/mol}$ (element in its standard state)
$\Delta H^\circ_f (\text{CO}_2(g)) = -393.5 \, \text{kJ/mol}$
$\Delta H^\circ_f (\text{H}_2\text{O}(l)) = -285.8 \, \text{kJ/mol}$
Step-by-Step Solution:
Write the formula for ΔHrxn∘:
$\Delta H^\circ_{\text{rxn}} = \sum (\Delta H^\circ_f \text{ of products}) - \sum (\Delta H^\circ_f \text{ of reactants})$
Substitute the values for the products:
Products: $\text{CO}_2(g)$ and $2\text{H}_2\text{O}(l)$
∑(ΔHf∘ of products)=[1⋅ΔHf∘(CO2(g))]+[2⋅ΔHf∘(H2O(l))]\sum (\Delta H^\circ_f \text{ of products}) = [1 \cdot \Delta H^\circ_f (\text{CO}_2(g))] + [2 \cdot \Delta H^\circ_f (\text{H}_2\text{O}(l))]∑(ΔHf∘ of products)=[1⋅ΔHf∘(CO2(g))]+[2⋅ΔHf∘(H2O(l))] =[1⋅(−393.5)]+[2⋅(−285.8)]= [1 \cdot (-393.5)] + [2 \cdot (-285.8)]=[1⋅(−393.5)]+[2⋅(−285.8)] =−393.5+(−571.6)=−965.1 kJ= -393.5 + (-571.6) = -965.1 \, \text{kJ}=−393.5+(−571.6)=−965.1kJ
Substitute the values for the reactants:
Reactants: CH4(g)\text{CH}_4(g)CH4(g) and 2O2(g)2\text{O}_2(g)2O2(g)
\sum (\Delta H^\circ_f \text{ of reactants}) = [1 \cdot \Delta H^\circ_f (\text{CH}_4(g))] + [2 \cdot \Delta H^\circ_f (\text{O}_2(g))]
=[1⋅(−74.8)]+[2⋅(0)]
=−74.8+0=−74.8 kJ
Calculate $\Delta H^\circ_{\text{rxn}}$:
$\Delta H^\circ_{\text{rxn}} = \sum (\Delta H^\circ_f \text{ of products}) - \sum (\Delta H^\circ_f \text{ of reactants})$
=−965.1−(−74.8)= -965.1 - (-74.8)
=−965.1+74.8=−890.3 kJ